How to climb - and why...
- Andreas
- Jul 4, 2020
- 6 min read
Updated: Jul 5, 2020
This article deals with the flight segment after the takeoff phase until reaching the cruise altitude. Industry practice is to draw the line between takeoff and climb at 1500 ft agl and to consider the climb until the first cruising level is reached [1].
During basic flight training, every pilot will come across two different climb methods “Vx” for best angle of climb and “Vy” for best rate of climb. Unfortunately, reality is much more complex and it would be quite an oversimplification to only consider these two possibilities. Throughout this article we will look at different climb methods, including the two “basic” ones in more detail in order to elevate our understanding of climbs.

Figure 1: Aerobatic aircraft performing a spectacular climb
Method 1: Vx (maximum climb angle)
It can be shown that the best angle of climb for a jet aircraft is achieved when it is operated at the highest thrust/weight ratio and simultaneously at the highest lift/drag ratio [2]. This is sometimes referred to as the “maximum excess thrust point”. For a pure turbojet aircraft the value of Vx as EAS remains fairly constant during the climb, until you reach the Mach limited region [2]. For a turbofan it tends to increase slightly until the aircraft becomes Mach limited [1]. The variation of Vx with altitude can be quite unique to a specific aircraft type and therefore a careful research is necessary if one is interested in a particular type.
Method 2: Vy (maximum climb rate)
The maximum rate of climb is achieved at the “maximum excess power point”. This is NOT the same as the maximum excess thrust point and always somewhat faster than Vx as Figure 2 demonstrates. For a typical transport category aircraft (turbofan) this speed (expressed as EAS) reduces slightly with increasing altitude [1]. Again, this follows a very specific pattern for each aircraft type. The rate of climb is of great interest to Air Traffic Control (ATC), as the separation between aircraft is largely achieved vertically.
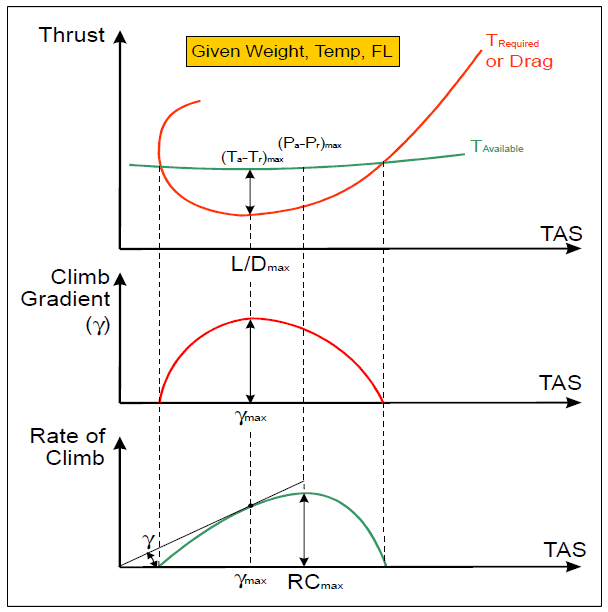
Figure 2: Relation between Vx and Vy for steady shallow climb, no Mach consideration [3]
Interesting point: Even if the exact variation of Vx and Vy as EAS with increasing altitude are quite specific for each aircraft type, it is generally correct to say that Vx and Vy approximate each other with increasing altitude and would theoretically become equal at the aircraft’s absolute ceiling [4] [5].
Method 3: Minimum climb fuel
One could suspect that the maximum rate climb at Vy (minimum time) will also yield the minimum fuel used for climb. Indeed the speed for minimum climb fuel is very close to Vy, but somewhat lower [1]. The reason for this is the dependence of fuel flow rate on airspeed. Imagine, you are climbing at Vy, and note the fuel flow rate. If you now slightly decrease the airspeed, there will be a reduction in fuel flow rate but a rather small change in climb rate (see Figure 2 and 3). So, as long as the reduction in fuel flow is more pronounced than the reduction in climb rate, this will slightly reduce climb fuel [1]. The concept is depicted in Figure 3.

Figure 3: Fuel flow (FF), rate of climb (ROC), speed for min. climb fuel (Vmcf), speed for best rate of climb (Vy). conceptual graph only.
Method 4: Minimum trip fuel
From an operator’s perspective, the flight does not end at the “top of climb”, so it is actually the minimum trip fuel that is of greater interest, rather than the minimum climb fuel. As it turns out, it is indeed more fuel efficient to use a somewhat shallower climb and displace the top of climb by several miles compared to the Vy climb profile [1]. Figure 4 depicts the various climb profiles in comparison to each other. Note, that Figure 4 is not to scale. It is obvious that the trip fuel starts to increase again, if the climb is too shallow, as the aircraft spends too much time in regions with a high fuel flow (see “min trip time” curve).

Figure 4: Conceptual overview of climb profiles for a turbofan transport aircraft, no Mach consideration, no performance degradation (not to scale)
Again, the profiles for “min climb fuel”, “min climb time” and “min trip fuel” are very close together and it can be very difficult to access such detailed data [2].
Method 5: Minimum cost
In a commercial world, it is actually not always beneficial to optimize fuel consumption. Sometimes it is more important to expedite the flight in order to meet some time constraints. The airline industry thus developed the parameter Cost Index (CI). The CI is the ratio of “cost of time” divided by “cost of fuel” [3]. Therefore, the guidance computers of a transport aircraft will calculate the optimum climb profile taking into account both the time-related cost and fuel-related cost [3]. This is obviously an individual number and thus an individual profile for each flight.
Method 6: Maximum gain in total energy
This method is a very special one and is only of practical interest for very high performance aircraft, such as a supersonic interceptor [2]. The idea is the following: Instead of looking at the altitude gain, cost and time, we could look at the gain in total energy (the sum of kinetic and potential energy). For a supersonic interceptor it is not very clever to climb very steeply just to arrive at the desired altitude with almost no kinetic energy [2]. Figure 5 introduces the concept of total energy height. The way to interpret this is as follows: If you are at 12000 m with zero speed, your energy height is 12000 m. If you now accelerate maintaining 12000 m, your total energy height increases.
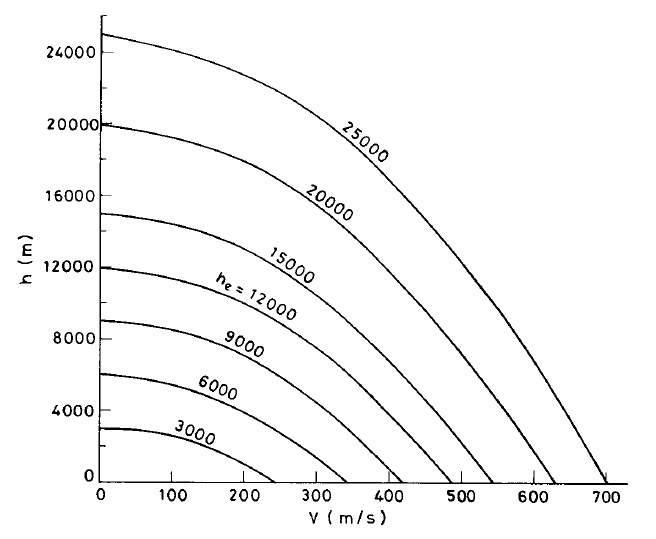
Figure 5: The concept of total energy height (he) [2]
This concept is of practical importance, if the sound barrier has to be penetrated during the climb [2]. A graph can be created, merging the total energy lines with “specific excess power” lines, in order to find where the most energy can be gained in the shortest amount of time (see Figure 6). This principle was originally introduced by Rutowski [6] and involves a descent (maintaining the total energy) in order to break the sound barrier and thereafter continue climb in supersonic regions with more specific excess power [2] [6].

Figure 6: “Rutowski climb profile”. The lines C1-C7 represent total energy levels. The lines b0-b5 represent specific excess power contours [2]
After all these fancy supersonic diagrams, let us get back to the subsonic transport aircraft of today:
Method 7: Reality
As always, even the best theory will face reality at some point. This also applies for climb schedules and often the theoretical optimum is exchanged for a practical regime taking into account real-world constraints such as airspace limitations and separation from other aircraft. Figure 7 depicts a more realistic climb schedule for a typical transport aircraft. Typically, a speed of 250 KIAS is maintained until FL100. Thereafter an acceleration is carried out to an economic climb speed (e.g. 300 KIAS). This is then maintained until the aircraft reaches the optimum climb Mach number, here Mach 0.78 [3]. An interesting point to note: When maintaining constant IAS, the TAS increases during climb. As the aircraft reaches the crossover altitude and starts to maintain a constant Mach number, TAS decreases again [3]. This creates a “spike” in the climb rate as the impulse (J) is converted into vertical speed [3].
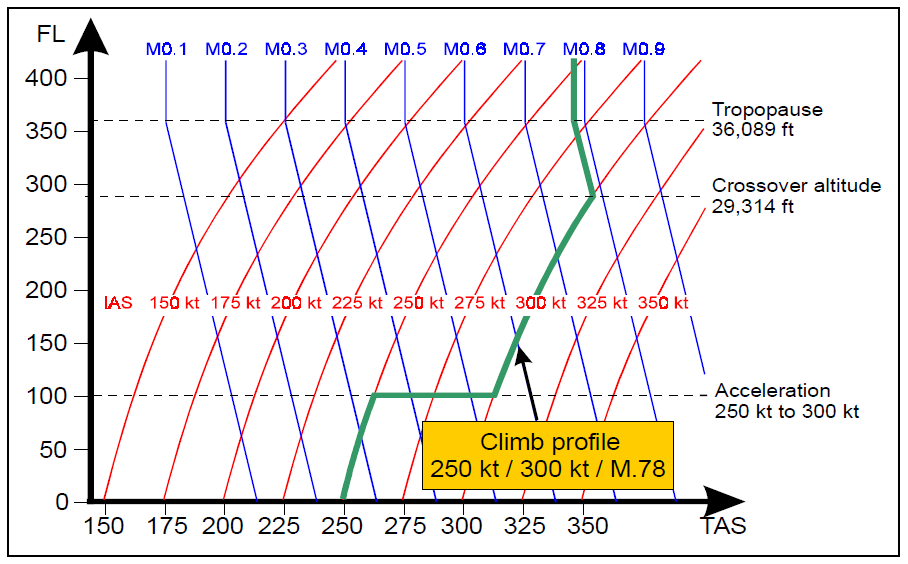
Figure 7: Typical climb profile in a real-world environment [3]
A word about guidance modes for climbing
There is a never-ending discussion amongst pilots, whether to use vertical speed mode or a mode that maintains the AOA if the performance decreases (e.g.: FLCH or Open Climb etc). From an engineering perspective, it is more efficient and much safer to maintain the AOA (FLCH/Open climb). If a pilot is forced by ATC to maintain a specific vertical speed, greatest care has to be taken not to decelerate towards regions of inefficient L/D ratios or even approach stall AOA!
This article dealt with different climb profiles. We shall see in another article, how different cruise profiles affect the range and economy of an aircraft.
Revision/20200705
References
[1] Blake and the Performance training group of Boeing, “Jet transport performance methods”, March 2009, Boeing
[2] OJHA, “Flight performance of aircraft”, 2005, AIAA
[3] AIRBUS, “Getting to grips with aircraft performance”, 2002, AIRBUS
[4] Swatton, “Aircraft performance theory for pilots”, 2005, Blackwell Science
[5] Swatton, “Principles of flight for pilots”, 2011, Wiley
[6] USAF Test pilot school, “Performance flight test phase”, volume 1, 1992, USAF
